Content:
What is a pt100 sensor?
Pt100 sensors are the most common type of platinum resistance thermometer. Often resistance thermometers are generally called Pt100 sensors, even though in reality they may not be the Pt100 type. Pt refers to that the sensor is made from Platinum (Pt). 100 refers to that at 0°C sensor has a resistance of 100 ohms (Ω).

 |  |
|---|---|
 |  |
 |
Characteristics of pt100:
Output: Resistance (Ω)
Range: -200 up to 850 °C
Relationship: Linear. Increases linearly with temperature
Advantages: High sensitivity with good range and highest accuracy possible
Disadvantages: Ohms must be converted to voltage. Non linear at high temperatures.
Theory:
our temperature range is from -10 to 30 °C, therefore pt100 value obtained from pt100 resistance table for this temperature will vary from 96.09 to 111.67 Ω. See
We would like to use full range of A/D, so we want the voltage out of bridge to start at 0V at -10 °C.
In order to do that, we make R3 equal to pt100 at it's lowest temperature. R3 = 96.09 Ω
Now, we have R3 = 96.09 Ω and pt100 = 111.67 Ω
From bridge should produce 1.203 mV at maximum temperature (30 °C).
When temperature is at maximum, pt100 = 111.67 Ω.
Assume Vs = 1V because sensitivity of Wheatstone bridge is small, so a small voltage is required.
Now, the Wheatstone bridge circuit should look like the following:

Calculations:
In this section, we are going to determine the resistance of R1 and R2 of the Wheatstone bridge; in order to do that we need
Wheatstone Bridge Equation:

Make R2 = R1 = R

Substitute Vo, Vs, pt100, and resistor values as follows:
Solve for R


Now we got a quadratic equation ready to be solved using quadratic formula:

Substituting values into quadratic formula.
a = 1
b = -12743.2
c = 10730.37
We get:
Wheatstone bridge circuit becomes:

We used R = 12742.35 Ω instead of R = 0.842 Ω. Note that using either values makes no difference in the output voltage form Wheatstone bridge.
Quantify Loading:
In this section we are going to calculate voltage out of bridge Vout,bridge and voltage out of differential amplifier Vout,amp without loading and with loading.

In order to do the calculations we need to simplify the Wheatstone bridge (left part of circuit) to become a single resistance value called Rbridge.


(4)

(1)

(2)
(3)

R and R3 are in parallel which gives Rx. Also, R and pt100 are in parallel which gives Ry. Both Rx and Ry are summed up in series which gives the total resistance in Wheatstone bridge (Rbridge).
The circuit then becomes:

We have to work out voltage out of bridge, Vout,bridge using the equation:

At 30 °C. (Remember our temperature range is from -10 to 30 °C)

OR
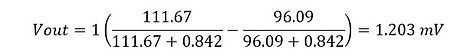
See that using either resistance values of R = 12742.35 or R = 0.842 gives the same output voltage.
Now, we have to work out voltage that goes in the Differential amplifier using the equation:


Amplifier gain = 5
If the voltage out of bridge Vout,bridge = 1.203 mV
Then Voltage out of amplifier Vout,amp = 5 × 1.203 mV = 6.015 mV
Due to loading, Voltage out of amplifier Vout,amp = 5 × 1.20 mV = 6mV



